By Steph Primiani, Director of STEM and Alicia Cuomo, Brown University Urban Education Policy Intern
A Protocol with Finesse
What if I told you that students can struggle, express high-level mathematical thinking, and be pushed to build on their present knowledge… all within 20 minutes?
Students and teachers at Blackstone Valley Prep are using a 20-minute Math Stories protocol to do just that.
In the last blog post, I discussed the Lesson Planning Protocol educators at Blackstone Valley Prep use each time they plan a Math Stories lesson. Teachers follow a four-step process: identify big ideas, anticipate multiple student representations, anticipate possible misconceptions, and plan targeted discussion questions in order to stamp a key point.
But what actually happens during those 20 minutes? What is the protocol for a Math Stories lesson?
The Math Stories Protocol
Take this story problem for example:
Molly walks 1 ½ miles each day for 5 days. Trevonte walks 2 ½ miles each day for 5 days. How much farther did Trevonte go than Molly in those 5 days?
Step 1: The Question 
The teacher reads the story problem twice to students. Pencils are down and students are asked to visualize, or make a mind-movie of, the story problem.
Students then write down the question they are trying to answer.
Step 2: Write Down the Information, Model and Solve
- Students write down the information they have and the information they need to solve the problem.
- Students model and solve the problem without any guidance.
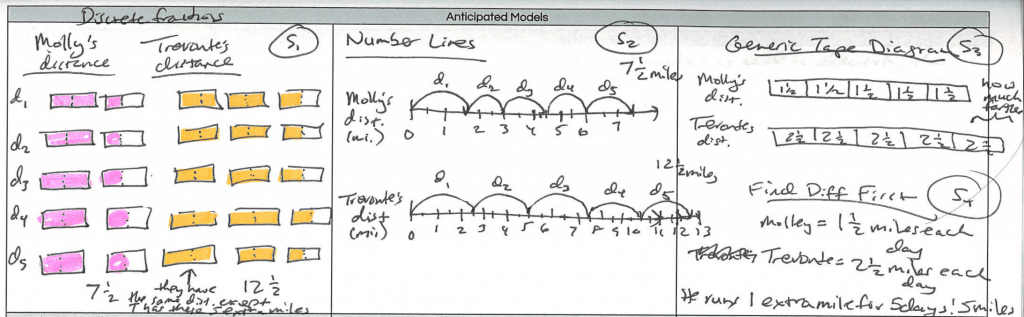
 The heart of Math Stories is that students model the problem using any representation strategy that makes sense to them. For example, students may choose to represent the number of miles Molly and Trevonte respectively walked over 5 days using a discrete fractions model, number lines, tape diagrams, or something else.
The heart of Math Stories is that students model the problem using any representation strategy that makes sense to them. For example, students may choose to represent the number of miles Molly and Trevonte respectively walked over 5 days using a discrete fractions model, number lines, tape diagrams, or something else.
An exemplar model:
- Has clearly labeled visual representations of Molly and Trevonte’s distances walked.
- Labels are specific so that other scholars can clearly see where the information from the problem is represented in the model.
- Solves the problem using the visual model, writing the algorithm that matches their model when it makes sense.
- Writes the final answer in a complete sentence.
Models:
Solve Strategies:
Students count/add to find the totals and subtract:
1 ½ + 1 ½ + 1 ½ + 1 ½ + 1 ½ = 7 ½
2 ½ + 2 ½ + 2 ½ + 2 ½ + 2 ½ = 12 ½
12 ½ – 7 ½ = 5
Trevonte walked 5 more miles than Molly.
Students multiply and subtract:
1 ½ x 5 = 7 ½
2 ½ x 5 =12 ½
12 ½ – 7 ½ = 5
Trevonte walked 5 more miles than Molly.
Students circle Trevonte’s “extra” miles and add:
Trevonte walks 1 more mile than Molly each day for 5 days. Trevonte walks 5 more miles than Molly.
Step 3: Turn-and-Talk
Students share their model and solution with a partner using math stories language:
“First, I… because in the problem… I solved by…”
Step 4: The Decision
 The teacher, who has been aggressively monitoring while students were modeling and solving, uses a data tracker to decide which of the 3 Ways to respond to student data.
The teacher, who has been aggressively monitoring while students were modeling and solving, uses a data tracker to decide which of the 3 Ways to respond to student data.
By gauging how much of the class is accurately modeling and solving, the teacher can have a targeted discussion that pushes students towards efficiency, using a more sophisticated strategy, and/or disproving a common misconception.
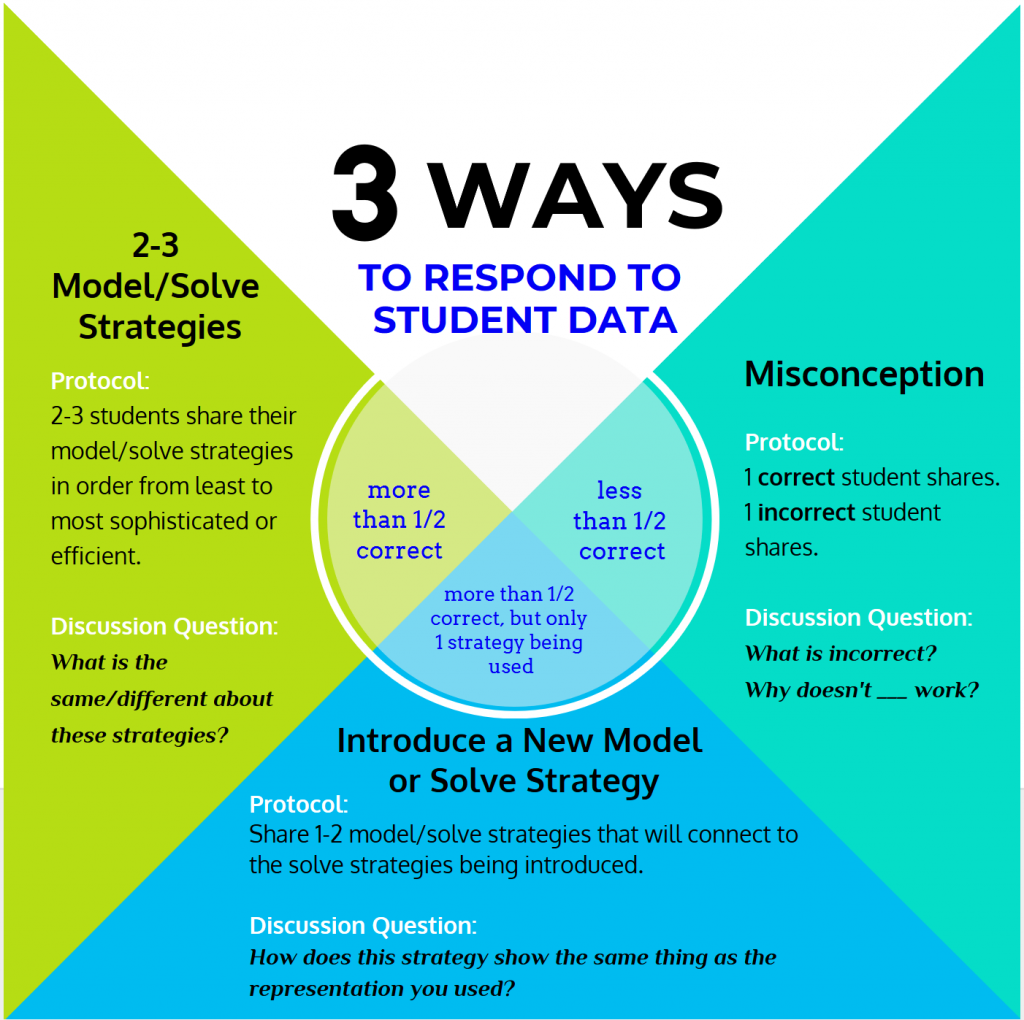
Students turn-and-talk to discuss the targeted question, and the teacher strategically facilitates a whole-class discussion, working to get students to arrive at a pre-planned exemplar response.
Finally, the teacher clearly states the Key Point and how it connects to the models and solution.
Step 5: Finish the Problem
Students use new knowledge from the discussion to either fix their solution or record a new way of solving.
Click here for a copy of the Math Stories Protocol template.
Student-Centered Discourse
The Math Stories protocol gives students the opportunity to grapple with mathematics in context without the training wheels of direct instruction or individual coaching.
One of the most valuable components is The Decision, in which educators use in-the-moment data to pose a meaningful discussion question that builds on students’ current knowledge. Using in-the-moment data is part of cognitively guided instruction, in which student thinking is the center of discourse rather than the teacher.1 Further, students of teachers who are knowledgeable about their thinking are associated with higher levels of achievement.2
View the Math Stories Protocol in action:
Want to Learn More?
In the next few posts, we will explore the importance of visualization and the power of learning from your peers.
Have questions? Reach out to BVP’s Director of STEM, Steph Primiani at sprimiani@blackstonevalleyprep.org and follow me on Twitter @stephprimiani
Resources
1 Clarke, Doug. “The Changing Role of the Mathematics Teacher,” 1997.
2 Carpenter et al. “Cognitively Guided Instruction: A Research-Based Teacher Professional Development Program for Elementary School Mathematics,” 2000.


